Cone

Definition

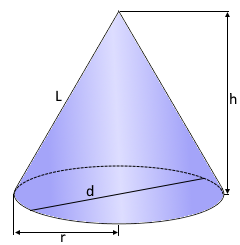
A cone is a three-dimensional geometric shape that tapers from a flat base (often but not necessarily circular) to a point called the apex or vertex.

Properties

• A cone is a three-dimensional geometric body with a round base.

• The side surface slopes upwards from the edge of the base and forms a point

• If the point is above the center of the base, the cone is straight. If the point deviates from the center of the base, it is called an oblique cone.

Formeln

The following formulas refer to the calculation of a straight cone

Radius

\(\displaystyle r=\sqrt{\frac{A}{ π}}\)

Diameter

\(\displaystyle d=\sqrt{\frac{4·A}{π}}\) \(\displaystyle \ \ \ =2· r\)

Height

\(\displaystyle h=\sqrt{L^2-r^2}\) \(\displaystyle \ \ \ =\frac{3·V}{A}\)

Lateral height

\(\displaystyle L=\sqrt{h^2+r^2}\)

Lateral surface

\(\displaystyle S_I=\frac{d·π·L}{2}\) \(\displaystyle \ \ \ =\frac{P· L}{2}\)

Surface

\(\displaystyle S=S_I+A\)

Volume

\(\displaystyle V=\frac{A·h}{3}\) \(\displaystyle \ \ \ =\frac{r^2· h}{3}\)

Cone online calculator →