Resistors in Series
Description how to calculate resistors in series
When multiple resistors are connected in a line where the current flows through them in sequence, we call it a series connection of resistors.
Total resistance
The total resistance results from the addition of the individual resistors.
\(R_{ges} = R_1+R_2+R3\)
Current
The flowing current through the individual resistors is equal and corresponds to the total current of the circuit.
\(I_{ges}=R_1+R_2+R_3\)
\(\displaystyle I=\frac{U}{R_{ges}}\)
Voltage
The total voltage across the total resistance is the sum of the individual voltages at the individual resistors
\(U=U_1+U_2+U_3\)
The applied total voltage is divided by the individual resistances in proportion to their values.
\(\displaystyle \frac{U_{ges}}{R_{ges}}=\frac{U_1}{R_1}=\frac{U_2}{R_2}=\frac{U_3}{R_3}\)
From this, the formula for a single voltage can be derived.
\(\displaystyle U_1=\frac{R_1·U_{ges}}{R_{ges}}\)
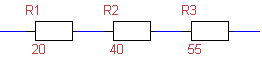
Example
We calculate the total resistance, the current and the individual voltages at the resistors. The total voltage is given with \(230\) Volt
\(\displaystyle R_{ges}=R_1+R_2+R_3=20+40+55=115Ω\)
\(\displaystyle I=\frac{U}{R_{ges}}=\frac{230}{115}=2A\)
\(\displaystyle U_1=R_1·I=20·2=40V\)or\(\displaystyle U_1=\frac{R_1·U_{ges}}{R_{ges}}=\frac{20·230}{115}=40V\)
\(\displaystyle U_2=R_2·I=40·2=80V\)or\(\displaystyle U_2=\frac{R_2·U_{ges}}{R_{ges}}=\frac{40·230}{115}=80V\)
\(\displaystyle U_3=R_3·I=55·2=110V\)or\(\displaystyle U_3=\frac{R_3·U_{ges}}{R_{ges}}=\frac{55·230}{115}=110V\)