Calculate RL Parallel Circuit
Calculator and formulas for calculating the parameters of an RL parallel circuit
RL Parallel Circuit Calculator
RL Parallel Circuit
The calculator calculates current, power, apparent and reactive resistance in the parallel connection of a resistor and an inductor. Enter the values for inductor, resistor, frequency and voltage.
Circuit Diagram & Parameters
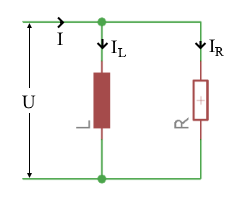
Parameter Legend
| I | Total current |
| IR | Current through the resistor |
| IL | Current through the inductor |
| XL | Inductive reactance |
| Z | Impedance (total resistance) |
| Y | Admittance (apparent conductance) |
| P | Real power |
| Q | Reactive power |
| S | Apparent power |
| φ | Phase angle |
|
|
|
|
Example Calculations
Practical Calculation Examples
Example 1: Low Frequency
Given: L = 100 mH, R = 100 Ω, f = 50 Hz, U = 230 V
Example 2: Audio Frequency
Given: L = 10 mH, R = 8 Ω, f = 1 kHz, U = 12 V
Example 3: High Frequency
Given: L = 1 µH, R = 50 Ω, f = 100 MHz, U = 5 V
Important Conversions
Frequency units:
Power units:
RL Parallel Circuit - Theory and Formulas
What is an RL Parallel Circuit?
In an RL parallel circuit, an ohmic resistor R and an inductance L are connected in parallel. The same voltage is applied to both components, but the current is distributed according to the respective resistance values. The total current is the geometric sum of the partial currents.
Calculation Formulas
Current Triangle
Total Current
Geometric addition of partial currents
Active Current
Current through the ohmic resistor
Reactive Current
Current through the inductance
Phase Angle
Phase shift between U and I
Conductance Triangle
For parallel circuits, it's often easier to work with conductances:
Admittance
Total conductance of the circuit
Conductance
Active conductance (reciprocal of resistance)
Susceptance
Reactive conductance of the inductance
Impedance (Total Resistance)
Total Impedance
Parallel connection of R and L
Reactance
Frequency-dependent reactance
Power Triangle
Apparent Power
Total power of the circuit
Real Power
Usable power in the resistor
Reactive Power
Oscillating power in the inductance
Power Factor
Ratio of real to apparent power
Practical Applications
Filters & Crossovers:
Motor Circuits:
Measurement Technology:
Behavior at Different Frequencies
Frequency-Dependent Behavior
- Low frequencies (f → 0): XL → 0, inductor acts like short circuit
- Medium frequencies: XL ≈ R, both branches contribute to current
- High frequencies (f → ∞): XL → ∞, resistor dominates
- Resonance: Does not occur in RL parallel circuits (only in RLC)
- Time constant: τ = L/R determines transient behavior
Design Guidelines
Important Design Aspects
- Current distribution: At low frequencies, more current flows through the inductor
- Losses: Power losses only occur in the resistor
- Phase angle: Current leads voltage (inductive)
- Reactive power: Inductor stores and returns energy
- Power quality: Parallel connection improves power factor
- Short-circuit behavior: Inductor limits current change