Triple Product Calculator
Online calculator for calculating the vector triple product
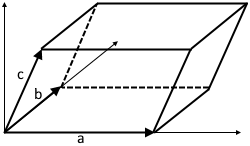
This function calculates the triple product of three vectors. The triple product is used to calculate the volume that is spanned by three vectors.
To calculate, enter the values of the three vectors, then click on the 'Calculate' button
Empty fields are evaluated as 0.
|
Formulas and examples
The triple product is used to calculate the volume that is spanned by three vectors.
1. Calculate triple product via cross product and dot product
\(\displaystyle triple product = (\vec{a} \times \vec{b})·\vec{c} \) \(\displaystyle = \left( \left[\matrix{a_1\\a_2\\a_3}\right] \times \left[\matrix{b_1\\b_2\\b_3}\right]\right) ·\left[\matrix{c_1\\c_2\\c_3}\right] \)
Example
\(\displaystyle \vec{a}=\left[\matrix{1\\1\\1}\right] \; \vec{b}=\left[\matrix{2\\1\\3}\right] \;\vec{c}=\left[\matrix{6\\0\\-2}\right] \)
Calculate cross product
\(\displaystyle \;\;\; \left[\matrix{a_1\\a_2\\a_3}\right] \times \left[\matrix{b_1\\b_2\\b_3}\right] =\left[\matrix{a_2·b_3-a_3·b_2\\a_3·b_1-a_1·b_3\\a_1·b_2-a_2·b_1}\right] \)
\(\displaystyle = \left[\matrix{1\\1\\1}\right] \times \left[\matrix{2\\1\\3}\right] =\left[\matrix{1·3-1·1\\1·2-1·3\\1·1-1·2}\right] =\left[\matrix{2\\-1\\-1}\right]\)
Calculate dot product
\(\displaystyle \left[\matrix{x_1\\x_2\\x_3}\right] \cdot \left[\matrix{y_1\\y_2\\y_3}\right] \) \( = x_1\cdot y_1 + x_2\cdot y_2 +x_3\cdot y_3\)
\(\displaystyle \left[\matrix{2\\-1\\-1}\right] \cdot \left[\matrix{6\\0\\-2}\right] \) \( = 2\cdot 6 + (-1)\cdot 0 +(-1)\cdot(-2)\) \(\displaystyle = 12 +0+2=14\)
2. Calculate the triple product using a matrix
The triple product can also be calculated using the determinant of a matrix.
\(\displaystyle D=\left[\matrix{a_1&b_1&c_1\\a_2&b_2&c_2\\a_3&b_3&c_3} \right]\)
\(\displaystyle D=\left|\matrix{1&2&6\\1&1&0\\1&3&-2}\right|\)
\(\displaystyle V= 1\cdot1\cdot(-2)+2\cdot0\cdot1 +6\cdot1\cdot3\) \(\displaystyle + 6\cdot1\cdot1 -1\cdot0\cdot3 -2\cdot1\cdot(-2)=14\)
|
|
Vector Functions
Addition • Subtraction • Multiplication • Scalar Multiplication • Division • Scalar Division • Dot Product • Cross Product • Interpolation • Distance • Distance Squaret • Normalization • Reflection • Magnitude • Squared-Magnitude • Triple-Product|
|