Pentakis Dodecahedron Calculator

Calculator and formulas for calculating a pentakis dodecahedron

The Golden Ratio Marvel - 60 Triangular Faces in Perfect Harmony!

Pentakis Dodecahedron Calculator

The Pentakis Dodecahedron

A Pentakis Dodecahedron is a Catalan solid with 60 isosceles triangular faces - dual to the truncated icosahedron.

Enter Known Parameter
Which parameter is known?
Value of the selected parameter
Pentakis Dodecahedron Calculation Results
Base Length a:
Leg Length b:
Surface Area A:
Volume V:
Circumradius RK:
Inradius RI:
Pentakis Dodecahedron Properties

The Golden Ratio Marvel: Dual to the truncated icosahedron

60 Triangular Faces 90 Edges 32 Vertices Golden Ratio

Pentakis Dodecahedron Structure

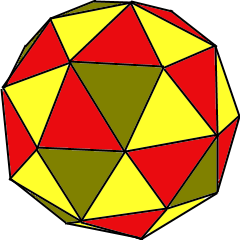
Pentakis Dodecahedron

The golden ratio marvel with 60 triangular faces.
Dual to the truncated icosahedron.

What is a Pentakis Dodecahedron?

A Pentakis Dodecahedron is a beautiful Catalan solid with icosahedral symmetry:

  • Definition: Polyhedron with 60 isosceles triangular faces
  • Faces: Each face is an isosceles triangle
  • Dual: To the truncated icosahedron (soccer ball)
  • Vertices: 32 vertices with different coordination
  • Edges: 90 edges in two lengths
  • Symmetry: Icosahedral symmetry group

Geometric Properties of the Pentakis Dodecahedron

The Pentakis Dodecahedron exhibits beautiful icosahedral geometric properties:

Basic Parameters
  • Edge Lengths: Two different edge lengths a (base) and b (leg)
  • Faces: 60 congruent isosceles triangles
  • Euler Characteristic: V - E + F = 32 - 90 + 60 = 2
  • Dual Form: Truncated icosahedron (soccer ball)
Golden Properties
  • Catalan Solid: Dual to Archimedean solid
  • Triangular Faces: Each face is an isosceles triangle
  • Golden Ratio: Proportions contain φ and √5
  • Icosahedral Symmetry: 120 symmetry operations

Mathematical Relationships

The Pentakis Dodecahedron follows mathematical laws based on the golden ratio:

Volume Formula
V ≈ 9.39·a³

Golden ratio formula with √5 terms. Coefficient ≈ 9.39 from icosahedral geometry.

Surface Area Formula
A ≈ 21.98·a²

Sum of 60 isosceles triangles. Golden ratio √5 geometry.

Applications of the Pentakis Dodecahedron

Pentakis Dodecahedra find applications in golden ratio based structures:

Scientific Research
  • Icosahedral crystallography
  • Viral capsid structures
  • Fullerene chemistry
  • Golden ratio studies
Engineering
  • Geodesic dome design
  • Soccer ball manufacturing
  • Icosahedral antennas
  • Sports equipment design
Education
  • Golden ratio education
  • Icosahedral symmetry studies
  • Catalan solid demonstrations
  • Mathematical art projects
Art & Design
  • Golden ratio sculptures
  • Icosahedral art installations
  • Architectural ornaments
  • Decorative geometric forms

Formulas for the Pentakis Dodecahedron

Base Length (a)
\[a = \frac{38 \cdot b}{3(9+\sqrt{5})}\] \[a \approx 1.27 \cdot b\]

Base length in terms of leg length with √5

Leg Length (b)
\[b = \frac{3a(9+\sqrt{5})}{38}\] \[b \approx 0.887 \cdot a\]

Leg length relationship with golden ratio

Surface Area (A)
\[A = \frac{15a^2\sqrt{413+162\sqrt{5}}}{19}\] \[A \approx 21.98 \cdot a^2\]

Surface area with complex √5 dependencies

Volume (V)
\[V = \frac{15a^3(23+11\sqrt{5})}{76}\] \[V \approx 9.394 \cdot a^3\]

Volume with golden ratio coefficients

Circumradius (RK)
\[R_K = \frac{a(3+\sqrt{5})}{4}\] \[R_K \approx 1.31 \cdot a\]

Circumradius with golden ratio factor

Inradius (RI)
\[R_I = \frac{3a}{2}\sqrt{\frac{81+35\sqrt{5}}{218}}\] \[R_I \approx 1.28 \cdot a\]

Inradius with complex √5 dependency

Isosceles Triangle Properties
60 Triangles
All isosceles and congruent
Two Edge Types
Base (a) and legs (b)
Golden Ratio
All proportions contain √5

Each triangle has two equal legs (b) and one base (a) in golden ratio proportions

Calculation Example for a Pentakis Dodecahedron

Given
Base Length a = 10

Find: All properties of the golden ratio marvel

1. Surface Area Calculation
\[A = 21.98 \times 10^2\] \[A = 21.98 \times 100\] \[A = 2198\]

The surface area is 2198 square units

2. Volume Calculation
\[V = 9.394 \times 10^3\] \[V = 9.394 \times 1000\] \[V = 9394\]

The volume is 9394 cubic units

3. Leg Length
\[b = 0.887 \times 10 = 8.87\]

The leg length is 8.87 units

4. Radii
\[R_K = 1.31 \times 10 = 13.1\] \[R_I = 1.28 \times 10 = 12.8\]

Circumradius: 13.1, Inradius: 12.8 units

5. The Golden Ratio Marvel
Base Length a = 10.0 Leg Length b = 8.87 Surface Area A = 2198
Volume V = 9394 Circumradius RK = 13.1 Inradius RI = 12.8
60 Triangular Faces ⭐ Golden Ratio ⚽ Soccer Ball Dual

The golden ratio marvel with perfect icosahedral symmetry

The Pentakis Dodecahedron: The Golden Ratio Marvel

The Pentakis Dodecahedron represents one of the most beautiful and mathematically elegant among the Catalan solids, embodying the perfect harmony of icosahedral symmetry and golden ratio mathematics. With its 60 congruent isosceles triangular faces, it demonstrates the remarkable way that the golden ratio φ = (1+√5)/2 can organize three-dimensional space into forms of extraordinary beauty and mathematical precision. As the dual to the truncated icosahedron (the familiar soccer ball), it transforms the complex vertex arrangements of its Archimedean partner into a uniform pattern of triangular faces, each governed by the same golden ratio relationships that appear throughout nature and art.

The Golden Ratio Foundation

The Pentakis Dodecahedron showcases golden ratio mathematics:

  • 60 Isosceles Triangles: Each face has two equal legs and one base in golden proportions
  • Icosahedral Symmetry: 120 symmetry operations of the highest three-dimensional order
  • Golden Ratio Proportions: All relationships involve φ and √5
  • Soccer Ball Dual: Dual to the most famous truncated polyhedron
  • Two Edge Types: Base length (a) and leg length (b) in golden ratio
  • Perfect Harmony: 32 vertices arranged in icosahedral perfection
  • Natural Connections: Found in viral structures and natural forms

Catalan Heritage and Icosahedral Duality

Catalan Excellence

As one of the most symmetric Catalan solids, the Pentakis Dodecahedron exemplifies the principle of duality at its finest. It shows how the complex face arrangements of the soccer ball transform into elegant triangular uniformity.

Icosahedral Duality

The duality with the truncated icosahedron creates a fascinating relationship: the familiar soccer ball pattern of pentagons and hexagons becomes a beautiful arrangement of 60 identical triangles.

Golden Harmony

With 60 faces (5×12), it perfectly embodies the pentagonal nature of icosahedral geometry, where the number 5 and its multiples appear naturally through the golden ratio relationships.

Mathematical Beauty

The formulas demonstrate the elegant way golden ratio mathematics can describe complex three-dimensional forms, with √5 appearing naturally in all calculations.

The Golden Ratio in Three Dimensions

The Pentakis Dodecahedron is a masterpiece of φ-based geometry:

Golden Proportions

The ratio between base and leg lengths follows golden ratio mathematics: a/b ≈ 1.27, derived from the relationship 38/(3(9+√5)), showcasing how φ naturally appears in icosahedral constructions.

√5 Mathematics

All formulas contain √5 terms, reflecting the fundamental role of the golden ratio φ = (1+√5)/2 in icosahedral geometry and connecting this polyhedron to the deepest patterns in mathematics.

Triangular Perfection

Each of the 60 isosceles triangles has identical proportions determined by golden ratio relationships, creating a perfect balance between geometric complexity and mathematical elegance.

Natural Harmony

The icosahedral symmetry and golden ratio relationships make this polyhedron appear frequently in nature, from viral capsids to molecular structures, demonstrating deep natural principles.

Scientific and Natural Significance

The Pentakis Dodecahedron appears throughout nature and science:

  • Viral Biology: Many virus capsids follow this geometric pattern
  • Crystallography: Model for icosahedral crystal structures and quasicrystals
  • Fullerene Chemistry: Related to C60 buckyball structures
  • Sports Design: Mathematical basis for soccer ball construction
  • Geodesic Architecture: Foundation for geodesic dome design
  • Art and Design: Golden ratio aesthetics in three dimensions
  • Mathematical Education: Perfect example of icosahedral symmetry

Construction and Golden Precision

Golden Precision

Manufacturing requires precise implementation of golden ratio relationships. Each triangle must maintain exact proportions to preserve the icosahedral symmetry and golden ratio aesthetics.

Mathematical Accuracy

The irrational nature of √5 requires high-precision calculations, but the results are more manageable than other irrational constants, making accurate physical construction feasible.

Natural Inspiration

The prevalence of this form in nature provides inspiration for biomimetic design, where natural golden ratio structures inform technological applications.

Aesthetic Appeal

The golden ratio proportions create inherent aesthetic appeal, making physical models particularly beautiful and suitable for artistic and educational display.

Cultural and Educational Impact

Golden Ratio Education

As a three-dimensional manifestation of golden ratio mathematics, it provides an excellent teaching tool for understanding how φ appears in geometry beyond the familiar two-dimensional examples.

Soccer Ball Connection

The duality with the soccer ball creates immediate recognition and interest, making it an excellent entry point for exploring advanced geometric concepts through familiar objects.

Artistic Inspiration

Artists and designers frequently use this form for its inherent golden ratio beauty, creating sculptures and installations that embody mathematical harmony in three-dimensional space.

Scientific Wonder

Its appearance in viral structures and molecular forms demonstrates the profound connection between mathematical beauty and natural efficiency, inspiring both scientific research and philosophical reflection.

Summary

The Pentakis Dodecahedron stands as a perfect example of how the golden ratio can organize three-dimensional space into forms of extraordinary beauty and mathematical elegance. Its 60 isosceles triangular faces, arranged in perfect icosahedral symmetry and governed by golden ratio relationships, make it both a mathematical masterpiece and a natural wonder. From its role as the dual to the familiar soccer ball to its appearance in viral structures and molecular forms, it demonstrates the deep connections between mathematical beauty, natural efficiency, and aesthetic appeal. As a bridge between pure mathematics and natural science, the Pentakis Dodecahedron continues to inspire researchers, educators, artists, and anyone fascinated by the golden ratio's power to create perfect harmony in three-dimensional space.

Disdyakis Triacontahedron • Deltoidal Hexecontahedron • Deltoidal Icositetrahedron • Hexakis Octahedron • Pentagonal Icositetrahedron • Pentakis Dodecahedron • Rhombic Dodecahedron • Rhombic Triacontahedron • Tetrakis Hexahedron • Triakis Octahedron • Triakis Tetrahedron • Triakis Icosahedron