Triakis Octahedron Calculator
Calculator and formulas for calculating a triakis octahedron (trigonal trisoctahedron)
Triakis Octahedron Calculator
The Triakis Octahedron
A Triakis Octahedron is a Catalan solid with 24 isosceles triangular faces - dual to the truncated cube.
Triakis Octahedron Properties
The Octahedral Triangle Master: Dual to the truncated cube
Triakis Octahedron Structure
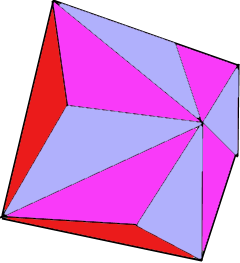
The octahedral triangle master with 24 faces.
Dual to the truncated cube.
What is a Triakis Octahedron?
A Triakis Octahedron is an elegant Catalan solid:
- Definition: Polyhedron with 24 isosceles triangular faces
- Faces: Each face is an isosceles triangle with two different edge types
- Dual: To the truncated cube
- Vertices: 14 vertices with different coordination
- Edges: 36 edges in two different lengths
- Symmetry: Octahedral symmetry group
Geometric Properties of the Triakis Octahedron
The Triakis Octahedron exhibits beautiful octahedral geometric properties:
Basic Parameters
- Edge Types: Octahedron edges (a) and pyramid edges (b)
- Faces: 24 congruent isosceles triangles
- Euler Characteristic: V - E + F = 14 - 36 + 24 = 2
- Dual Form: Truncated cube
Octahedral Properties
- Catalan Solid: Dual to Archimedean solid
- Triangular Faces: Each face is an isosceles triangle
- √2 Ratio: Proportions contain √2
- Octahedral Symmetry: 48 symmetry operations
Mathematical Relationships
The Triakis Octahedron follows elegant mathematical laws with √2:
Volume Formula
Simple formula with √2. Coefficient = 2-√2.
Surface Area Formula
Sum of 24 isosceles triangles. Complex √2 geometry.
Applications of the Triakis Octahedron
Triakis Octahedra find applications in cubic studies:
Scientific Research
- Cubic crystallography
- Crystal structure analysis
- √2 studies
- Mathematical symmetry
Engineering
- Octahedral structures
- Cubic design
- Geometric optimization
- Mathematical modeling
Education
- √2 ratio education
- Octahedral symmetry studies
- Geometry courses
- Mathematical demonstrations
Art & Design
- Octahedral sculptures
- √2 art installations
- Mathematical decorations
- Geometric pattern design
Formulas for the Triakis Octahedron
Pyramid Edge (b)
Pyramid edge in terms of octahedron edge with √2
Surface Area (A)
Surface area with complex √2 dependencies
Volume (V)
Volume with simple √2 coefficient
Midsphere Radius (RK)
Simple relationship - half the octahedron edge
Insphere Radius (RI)
Insphere radius with √2 dependency
Edge Relationship
The ratio between pyramid and octahedron edges
Isosceles Triangle Properties
All isosceles and congruent
Octahedron (a) and pyramid (b) edges
All proportions contain √2
Each triangle has one octahedron edge (a) and two pyramid edges (b) in √2 proportions
Calculation Example for a Triakis Octahedron
Given
Find: All properties of the octahedral triangle master
1. Surface Area Calculation
The surface area is 366 square units
2. Volume Calculation
The volume is 585.8 cubic units
3. Pyramid Edge
The pyramid edge is 5.858 units
4. Radii
Midsphere: 5.0, Insphere: 4.8 units
5. The Octahedral Triangle Master
The octahedral triangle master with perfect √2 symmetry
The Triakis Octahedron: The Octahedral Triangle Master
The Triakis Octahedron stands as one of the most elegant and mathematically refined among the Catalan solids, representing the perfect synthesis of octahedral symmetry and triangular geometry. With its 24 congruent isosceles triangular faces, each having two different edge lengths in √2 proportions, it demonstrates how the fundamental mathematical constant √2 can organize three-dimensional space into forms of extraordinary geometric harmony. As the dual to the truncated cube, it transforms the mixed face arrangements of its Archimedean partner into a uniform pattern of triangular faces, creating a polyhedron that serves as both a mathematical masterpiece and a natural expression of octahedral perfection.
The Octahedral Foundation
The Triakis Octahedron showcases octahedral √2 mathematics:
- 24 Isosceles Triangles: Each face has one octahedron edge and two pyramid edges
- Octahedral Symmetry: 48 symmetry operations of cubic crystallography
- √2 Proportions: All relationships involve √2
- Edge Diversity: Two different edge lengths in √2 ratio
- Simple Mathematics: Elegant formulas with √2 terms
- Cubic Heritage: Related to cube and octahedron
- Natural Perfection: Appears in crystalline structures
Catalan Heritage and Octahedral Duality
Catalan Excellence
As one of the elegant Catalan solids, the Triakis Octahedron perfectly demonstrates the principle of duality with the truncated cube, showing how mixed face arrangements become uniform triangular patterns.
Octahedral Duality
The duality with the truncated cube creates a fascinating relationship where the complex face types (triangles and octagons) of the dual become uniform isosceles triangles with consistent proportions.
Triangular Perfection
With 24 faces arranged in perfect octahedral symmetry, each triangle maintains √2 edge relationships, creating a form where mathematical perfection and geometric beauty converge.
Mathematical Beauty
The formulas demonstrate the elegant way √2 mathematics can describe complex three-dimensional forms, with √2 appearing naturally in all calculations and edge relationships.
The √2 Ratio in Triangular Form
The Triakis Octahedron is a masterpiece of √2-based geometry:
Edge Relationships
The ratio between pyramid and octahedron edges follows simple √2 mathematics: b = (2-√2)·a ≈ 0.5858·a, showcasing how √2 naturally appears in octahedral constructions.
√2 Mathematics
All formulas contain √2 terms, directly connecting to the fundamental relationship in cubic geometry. The elegant formulas show how √2 governs the polyhedron's properties.
Triangular Harmony
Each of the 24 isosceles triangles has identical proportions determined by √2 relationships, creating a perfect balance between geometric simplicity and mathematical elegance.
Octahedral Harmony
The octahedral symmetry and √2 relationships make this polyhedron appear in crystalline structures, demonstrating deep natural mathematical principles.
Summary
The Triakis Octahedron stands as a perfect example of how √2 and octahedral symmetry can combine to create forms of extraordinary mathematical elegance and geometric beauty. Its 24 isosceles triangular faces, each with edges in √2 proportions and arranged in perfect octahedral symmetry, make it both a mathematical masterpiece and a natural wonder. From its role in crystalline structures to its applications in symmetry studies, it demonstrates the deep connections between mathematical beauty, natural phenomena, and aesthetic perfection. As a bridge between pure mathematics and crystallographic reality, the Triakis Octahedron continues to inspire researchers, educators, artists, and anyone fascinated by √2's power to create perfect harmony in three-dimensional forms.