Truncated Cuboctahedron
Calculator and formulas for calculating a truncated cuboctahedron
This function calculates various properties of a truncated cuboctahedron. A truncated cuboctahedron has 12 square faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices, and 72 edges.
To perform the calculation, select the property you know and enter its value. Then click on the 'Calculate' button.
|
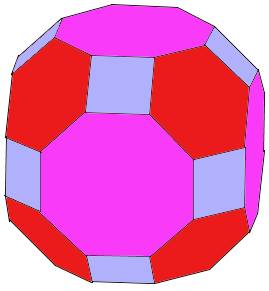
Truncated Cuboctahedron Formulas
Volume \(\small{V}\)
\(\displaystyle V= 2· a^3 · (11+7·\sqrt{2})\)
Surface area \(\small{S}\)
\(\displaystyle S= 12 · a^2 ·(2+\sqrt{2}+\sqrt{3})\)
Outer radius \(\small{r_c}\)
\(\displaystyle r_c=\frac{a·\sqrt{13+6·\sqrt{2}}}{2} \)
Midsphere radius \(\small{r_m}\)
\(\displaystyle r_m=\frac{a·\sqrt{12+6·\sqrt{2}}}{2}\)
Edge length \(\small{a}\)
\(\displaystyle a= \sqrt[3]{ \frac{V}{2 ·(11+7· \sqrt{2})}} \)
\(\displaystyle a= \sqrt{ \frac{S}{12·(2+\sqrt{2}+\sqrt{3})}} \)
\(\displaystyle a=\frac{2·r_c}{ \sqrt{13+6·\sqrt{2}}} \)
\(\displaystyle a= \frac{2 ·r_m}{\sqrt{12+6·\sqrt{2}}} \)