Kite Calculation
Calculator and formula for calculating the parameters of a kite
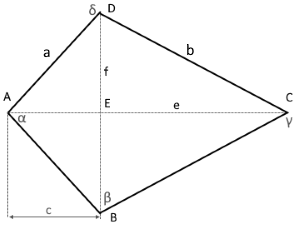
This function calculates the properties of a kite. A kite is a quadrilateral whose four sides can be grouped into two pairs of equal-length sides that are adjacent to each other.
For the calculation, enter the lengths of the two diagonals an e and f and the distance c. In result the angles are displayed in degrees.
|
Description
A kite is a quadrilateral that has two pairs of adjacent sides of equal length. A kite square also has the following properties:
- The diagonals e and f are perpendicular to each other.
- The diagonal e (from A to C) is the axis of symmetry.
- The diagonal f (from B to D) divides the kite into two isosceles triangles.
- The opposite angles in the corner points B and D are the same size.
Formulas for the kite
Side length (a)
\(\displaystyle a= \sqrt{ \left(\frac{f}{2}\right)^2 + c^2}\)
Side length (b)
\(\displaystyle b= \sqrt{ \left(\frac{f}{2}\right)^2 + (e-c)^2}\)
Area (A)
\(\displaystyle A=\frac{e · f}{2}\) \(\displaystyle =a · b · sin(β)\)
Perimeter (P)
\(\displaystyle P=2 · a + 2 · b\) \(\displaystyle =2 · (a+b)\)
Diagonal (e)
\(\displaystyle e= \sqrt{a^2+b^2-2 · a · b ·cos(β)}\)
Diagonal (f)
\(\displaystyle f= 2 · a · sin\left(\frac{α}{2}\right)\) \(\displaystyle = 2 · b · sin\left(\frac{γ}{2}\right)\)
Angle (α)
\(\displaystyle α = arccos\left(\frac{2 · a^2 - f^2}{2 · a^2} \right)\)
Angle (γ)
\(\displaystyle γ = arccos\left(\frac{2 · b^2 - f^2}{2 · b^2} \right)\)
Angle β
Angle δ
\(\displaystyle β \ \ = δ \ \ = arccos\left(\frac{a^2+ b^2 - e^2}{2 · a · b} \right)\)

|
|
Square • Rectangle • Golden Rectangle • Rectangle to Square • Rhombus, given varios parameter • Rhombus , given diagonal e, f • Parallelogram, given 2 sides and angle • Parallelogram area, given side and height • Trapezoid • Cyclic Quadrilateral • General Quadrilateral • Concave Quadrilateral • Arrowhead Quadrilateral • Crossed Square • Frame • Kite, given 2 diagonal and distance • Kite Area, given 2 diagonal • Half Square Kite • Right Kite"
|
|
|
|
|
|