Calculate rhombus
Calculator and formulas for the equal-sided rhombus
Rhombus calculator
The rhombus (diamond)
A rhombus is an equal-sided quadrilateral with four equal sides and opposite sides parallel.
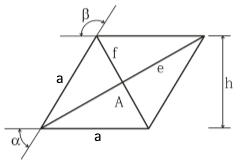
Rhombus structure
Rhombus properties
Special case: all sides equal, opposite angles equal
Rhombus with four equal sides a.
Diagonals are perpendicular to each other.
The rhombus: diamond-shaped perfection of equilateralness
The rhombus combines properties of a parallelogram with four equal sides:
- Four equal sides: |a| = |b| = |c| = |d|
- Parallelism: Opposite sides are parallel
- Supplementary angles: α + β = 180° (adjacent angles)
- Equal opposite angles: α = γ and β = δ
- Perpendicular diagonals: e ⟂ f at the center
- Double area formula: A = a×h = (e×f)/2
Special geometry of the rhombus
The geometric properties of the rhombus are unique among quadrilaterals:
Diagonals system
- Diagonals are perpendicular (e ⟂ f)
- Diagonals bisect at the center
- Diagonals bisect interior angles
- Area formula: A = (e × f) / 2
Angle properties
- Opposite angles are equal (α = γ)
- Adjacent angles are supplementary (α + β = 180°)
- Height h = a × sin(α) = a × sin(β)
- Two axes of symmetry (the diagonals)
Mathematics of rhombus calculation
The mathematical relations of the rhombus use trigonometry and geometry:
Trigonometric relations
- Height: h = a × sin(α) = a × sin(β)
- Area: A = a² × sin(α)
- Diagonals computed via cosine formulas
- Angle from height: α = arcsin(h/a)
Diagonal computation
- Side length: a = √((e/2)² + (f/2)²)
- e = 2a × cos(α/2), f = 2a × cos(β/2)
- Height relation: e = h/sin(α/2)
- Pythagorean relations for diagonals
Applications of the rhombus
Rhombi have many applications in nature, art and engineering:
Crystals & minerals
- Diamond crystal structure
- Rhombic crystal systems
- Calcite and other minerals
- Molecular lattice structures
Design & art
- Diamond logo design
- Rhombic patterns and ornaments
- Textile designs (argyle patterns)
- Architectural tiling designs
Engineering & construction
- Parallelogram linkages in vehicles
- Expanded metal and lattice structures
- Truss constructions
- Mechanical gear systems
Games & symbols
- Playing card diamond suit
- Traffic sign geometry
- Baseball diamond (field)
- Warning signs and pictograms
Formulas for the rhombus
Area A
Three ways to compute the area
Side length a
From area/height or diagonals
Height h
Height from area or trigonometrically
Perimeter P
Four equal sides
Diagonal e
Longer diagonal
Diagonal f
Shorter diagonal
Angle relations
Supplementary angles and trigonometric computation
Worked example for a rhombus
Given
Find: All parameters of the rhombus
1. Compute base parameters
Area and perimeter directly computable
2. Determine angles
Trigonometric angle calculation
3. Compute diagonals
Cosine formulas for both diagonals
4. Complete rhombus
Complete rhombus with diamond-shaped elegance!
The rhombus: diamond-shaped perfection between square and parallelogram
The rhombus, as an equal-sided parallelogram, unites parallelism and equal side lengths into a unique geometric form. As the diamond on playing cards, as a crystal structure in nature or as an elegant design element in architecture, the rhombus fascinates through its perfect balance between symmetry and dynamism, between stability and visual motion.
The mathematical elegance of the rhombus
The rhombus illustrates fascinating geometric principles:
- Dual identity: Parallelogram with four equal sides
- Perpendicular diagonals: e ⟂ f - unique among parallelograms
- Dual area formula: A = a×h = (e×f)/2 - two computation paths
- Trigonometric completeness: All parameters computable from two values
- Angle symmetry: α + β = 180°, α = γ, β = δ
- Diagonal bisection: Center divides both diagonals
The rhombus in nature and crystallography
In nature the rhombus appears in impressive variety:
Crystal systems
The rhombic crystal system shows rhombus-like structures in three dimensions. Calcite, gypsum and many other minerals crystallize in rhombic forms.
Molecular geometry
Benzene rings and other organic compounds show rhombic symmetries. Diamond crystal structure is based on rhombic building blocks.
Biological structures
Honeycomb structures, scale arrangements and plant patterns use rhombic geometries for optimal space utilization.
Geology
Basalt columns, sediment layers and tectonic structures often show rhombic fracture patterns and symmetries.
Cultural and symbolic meaning
The rhombus carries deep cultural and symbolic meanings:
- Playing card symbolism: Diamond suit as a symbol of wealth and materiality
- Heraldic tradition: Rhombi in coats of arms symbolize nobility and dignity
- Textile design: Argyle patterns and rhombic ornaments in Scottish traditions
- Traffic symbolism: Rhombic warning signs for increased attention
- Religious symbolism: Rhombi as symbols of earthiness and stability
- Modern aesthetics: Diamond logos as signs of quality and exclusivity
Technical applications of rhombus geometry
Engineering and technology use rhombus structures for optimal functionality:
Mechanical systems
Parallelogram linkages in vehicles use rhombus geometry for smooth force transmission. Scissor lifts use rhombic mechanics.
Structural applications
Expanded metal and mesh use rhombic patterns for optimal strength with minimal material.
Optical systems
Prism arrays and optical gratings use rhombic arrangements for light refraction and dispersion.
Electronics
Antenna arrays and sensor networks use rhombic geometries for optimal coverage and signal distribution.
Future perspectives of rhombus geometry
Modern technologies open new application fields for rhombus structures:
- Metamaterials: Rhombic unit cells for exotic optical properties
- Nano-technology: Molecular rhombi as building blocks for nano-machines
- Smart materials: Adaptive structures with variable rhombus geometry
- Robotics: Rhombic robot configurations for optimal mobility
- Architecture 4.0: Responsive facades with dynamic rhombic patterns
- Quantum computing: Rhombic qubit arrangements for error correction
Summary
The rhombus stands as a diamond-shaped symbol of geometric elegance and mathematical completeness. As an equal-sided parallelogram it unites symmetry with dynamism, stability with visual motion. Its unique properties - perpendicular diagonals, dual area formulas and trigonometric completeness - make it a fascinating subject of geometric study. From the diamond's crystal structure to cultural symbols and modern technical applications, the rhombus remains a timeless example of how mathematical perfection and practical functionality merge in harmonious unity. It reminds us that true beauty often lies in perfect balance - four equal sides that open infinite possibilities.
|
|