Spherical ring
Calculator and formulas for calculating a spherical ring
A ball ring is a solid ball that contains a cylindrical hole. It is bounded on the outside by a symmetrical spherical layer and on the inside by the lateral surface of a straight circular cylinder.
To calculate the spherical ring, enter the radius of the sphere and the radius of the hole a. Then click on the 'Calculate' button
|
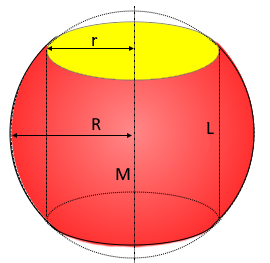
Spherical Ring Formulas
Ring volume (\(\small{V_R}\))
\(\displaystyle V_R=\frac{π·L^3}{6}\)
Cylinder height (\(\small{L}\))
\(\displaystyle L=2·\sqrt{R^2-r^2}\)
Cylinder volume (\(\small{V_C}\))
\(\displaystyle V_{C}=π·L· r^2\)
Ring surface (inside + outside) (\(\small{S}\))
\(\displaystyle S=4· π·(r+R)·\sqrt{R^2-r^2}\) \(\displaystyle \ \ \ =2· π·L ·(r+R)\)
You can find more information about the spherical ring in the tutorial
Round solids functions
Sphere • Spherical cap • Spherical sector • Spherical segment • Spherical ring • Spherical wedge • Spherical corner • Spheroid • Triaxial ellipsoid • Ellipsoid volume • Spherical shell • Solid angles • Torus • Spindle torus • Oloid • Elliptic Paraboloid