Regular Prism Calculator
Online calculator and formulas for calculating a regular prism
Regular Prism Calculator
The Regular Prism
A Regular Prism is a prismatic body with parallel regular polygonal faces.
Regular Prism Properties
The perfect prismatic form: Two parallel regular polygonal faces
Regular Prism Structure
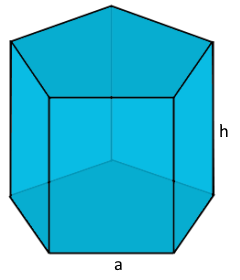
The perfect prismatic form with parallel faces.
Uniform height throughout.
What is a Regular Prism?
A Regular Prism is a fundamental prismatic solid:
- Definition: Prism with regular polygonal bases
- Parallel faces: Two identical parallel polygons
- Side faces: n congruent rectangles
- Vertices: 2n vertices total
- Edges: 3n edges (n+n+n)
- Uniformity: Constant cross-section
Geometric Properties of Regular Prisms
The Regular Prism demonstrates remarkable geometric properties:
Basic Parameters
- Base faces: 2 regular n-gons
- Side faces: n rectangles
- Euler characteristic: V - E + F = 2n - 3n + (n+2) = 2
- Cross-section: Constant throughout height
Special Properties
- Translation symmetry: Along the height axis
- Rotation symmetry: n-fold around central axis
- Reflection symmetry: Multiple planes
- Right angles: Between bases and sides
Mathematical Relationships
The Regular Prism follows elegant trigonometric laws:
Volume Formula
Simple multiplication of base area and height. Clean and elegant.
Surface Formula
Sum of two bases and rectangular sides. Elegant geometric form.
Applications of Regular Prisms
Regular Prisms find applications in various fields:
Architecture & Construction
- Building columns and pillars
- Structural beams
- Foundation elements
- Architectural details
Science & Engineering
- Crystal structures
- Optical prisms
- Mechanical components
- Packaging design
Education & Teaching
- Geometry instruction
- Volume calculations
- 3D geometry studies
- Mathematical demonstrations
Art & Design
- Sculptural works
- Decorative elements
- Furniture design
- Geometric patterns
Formulas for Regular Prism
Surface Area (S)
Surface area with tangent formula
Volume (V)
Volume = Base area × Height
Base Area (A)
Area of regular n-gon base
Height (h)
Height from volume and base
Regular Prism Parameters
2 regular n-gons
n rectangles
2n vertices
3n edges
The (n) stands for the number of vertices of the base polygon
Calculation Example for a Regular Prism
Given
Find: Height of the pentagonal prism
1. Base Area Calculation
For n=5, a=5:
\[A = \frac{5 \cdot 25}{ 4 \cdot \tan(36°)}\] \[A ≈ \frac{125}{4 \cdot 0.727}\] \[A ≈ 43.01\]The base area is approximately 43.01 square units
2. Height Calculation
Using V = A × h:
\[h = \frac{V}{A} = \frac{140}{43.01}\] \[h ≈ 3.26\]The height is approximately 3.26 length units
3. The Pentagonal Prism
The pentagonal prism with perfect parallel symmetry
The Regular Prism: The Perfect Prismatic Form
The Regular Prism is one of the most fundamental and elegant geometric solids, representing the perfect balance between simplicity and structural integrity. With its two parallel regular polygonal faces connected by rectangular sides, the regular prism embodies the essence of prismatic geometry. The mathematical beauty lies in the simple yet powerful relationships between its dimensions, making it both theoretically fascinating and practically invaluable in countless applications.
The Geometry of Perfection
The Regular Prism demonstrates the elegance of parallel geometry:
- Parallel bases: Two identical regular polygons
- Uniform cross-section: Constant throughout the height
- Right angles: Perfect perpendicular relationship
- Translation symmetry: Invariant under translation
- Rotation symmetry: n-fold rotational symmetry
- Reflection symmetry: Multiple reflection planes
- Structural stability: Inherently strong form
Mathematical Elegance
Simple Formulas
The formulas for regular prisms are elegant in their simplicity, using basic trigonometric functions to relate all geometric properties.
Scalable Design
From triangular to highly polygonal bases, regular prisms scale beautifully while maintaining their fundamental properties.
Practical Applications
The simple geometry makes regular prisms ideal for construction, manufacturing, and countless engineering applications.
Universal Form
Regular prisms appear throughout nature and human design, from crystal structures to architectural elements.
Summary
The Regular Prism stands as a testament to the power of geometric simplicity. Its perfect parallel faces, elegant mathematical relationships, and inherent structural strength make it one of the most important shapes in mathematics, engineering, and design. From ancient architecture to modern manufacturing, the regular prism continues to serve as a foundation of geometric thinking and practical application. Its beauty lies not in complexity, but in the perfect harmony of simple geometric principles working together to create an endlessly useful and aesthetically pleasing form.
|
|
Tetrahedron • Cube • Octahedron • Dodecahedron • Icosahedron